Tri Satyo S.P
Independent Seismic Consultant
For Designing Land 3D Seismic Survey
BASIC CONCEPT
There are some new aspects to the survey design in three dimensions relative to 2-D surveys. The 2-D surveys are as linear as the terrain allows. Source and receiver are normally in-line with each other. Arrays may be multidimensional but most often are also in the line of survey. For 3-D surveys this is seldom the case.
The 3-D survey also includes multiple source lines as well as multiple receiver lines, and it is possible to record two source lines. The arrays also may respond in less predictable manner as they are not necessarily in line with either the source or receiver locations. If the survey is planned to acquire a good directional range of offsets, the arrays will see oncoming wavefront from a number of angles. This will require a more sophisticated analysis of the array effect. Use of the patterns and other multi-azimuth array patterns is sometimes practiced.
The accent of 2-D lines is on the fold coverage and offset range. For 3-D survey, the fold may be less but the azimuth range is added to the offset range as a parameter. If structure is complex, the good azimuthal range become important. In fig-1. There are some arbitrary number of seismic traces in a bin. For velocity analysis the bin needs to contain a range of offsets. The range of azimuth in a bin is also a consideration. The concept of azimuths also is a new factor in 3-D survey.
The azimuthal property is not significant when the geology features only gentle dips and lateral consistency. The effect of dip is to increase apparent velocity. Thus the velocity analysis must have an azimuthal property. The imaging of complex structure is also improved by surveys with a good range of azimuths.
The Fresnel zone takes on some new characteristics in 3-D. Generally, even in 2-D, this important concept is given a small amount of attention. As the target sizes historically decrease in the size the zone become more important.
Essentially, the theoretical point source expands as it propagates in depth, “illuminating” a circular area at vertical incidence. In the seismic context this is the reflecting surface constructively contributing to the reflection. A good approximation to the radius of the zone is :
RF = ( V-avg/2) * (T/F)1/2
which show that the zone increases in radius with depth but decrease with higher frequency wavefronts. Migration serves to reduce the zone to some minimal size when accurately done and the data fits the assumption.
PRELIMINARY BASIC PARAMETERS
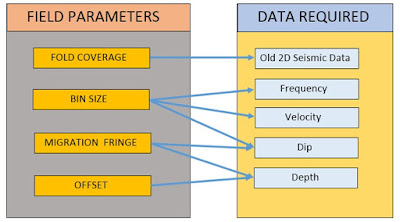
There are some parameters that need to be estimated as an input to designing 3D survey. The physics and concepts are somewhat independent of whether the survey is to have two or three dimensions or just involve some modification to their calculation.
1. Offset
The imaging of shallow, target, and deep horizons still requires certain offsets of source and receiver. The calculation and direction may be different but the rule is :
Offset = depth of target horizon
2. Fold
The fold required for noise compression is a function of the local S/N conditions. This translates in 3D to the number of trace in bin. Because of the extra focusing by migration and the flexibility of binning, fold can be less than required 2-D survey.
3. Frequency
The temporal frequency required Is not much different from that for 2-D survey. The rule for the resolution of layer of given thicknesses are best determined by modeling. The general rule is that the resolution of thin bed requires it to be sampled twice with a quarter wavelength of the highest frequency.
4. Migration
At this point it is relevant to remember that when dipping beds are in the preliminary model of the survey the extent of the survey must be increased. The tangent of the angle of maximum dip modifies the areal extent of the survey.
A 3D DESIGN SEQUENCES
There are so many ways to begin and complete a survey design. The specific sequence of steps that follow are general guidelines. The information gathering, modeling, and dimensionally independent parameters, such as offset range, are presumed to have been computed as previously describe. We must determine the offset ranges, temporal frequency, required fold, bin size, and available field equipment capacity. Less direct variables such as survey size adjustment for migration and any azimuthal requirements are also presumed to be ready. Another assumption is that the 3D software can cope with the template you have in mind. A summary of the proposed sequence for developing in design script is :
Step-1
Determine the subsurface bin size. Twice the chosen bin size is source and receiver station spacing.
Step-2
Compute the number of source stations per square kilometer required to achieve fold with the available equipment. The number of stations per square kilometer allow computation of source line spacing.
Step-3
Compute a receiver line spacing.
Step-4
Find the number of receiver lines allow by field equipment constrained by the required offset ranges. The result is the template.
Step-5
Decide on the x and y roll along.
Step-6
Allow for obstacles and run analyses of the script for offset distribution in the bins, ranges of offset in the bins, and azimuthal properties of the bin.
Step-7
Estimate time and costs of the script and iterate until attributes, costs and time are satisfied. Write the shooting script. Prepare to make more adjustments when the survey is begun. Often conditions found in the field requires changes in the shooting script.
Fig-2. A Schematic of 3D Design
Group and Source Interval
The CDP bin size is the spacing between the stacked traces in the seismic cube and represents the limit of spatial resolution. Therefore, all factors that limit resolution must be larger than the CDP size.
Group Interval
The group interval is the basic sampling of the earth by the survey. The custom is to compute this interval so that the recording data is clear from aliasing effect. The formula for finding the largest spatial sampling will prevent aliasing is :
G = 0.5*(Va/Fmax) / sin θx
Or inline bin size, ΔX = 0.25*(Va/Fmax) / sin θx
Where G is group interval, Va is average velocity, Fmax is maximum frequency of the objective horizon and θx is dip of the horizon inline direction. Example : a 65 Hz wave travelling in a medium with dip 30 degree which has propagation velocity of 2150 m/s has a Group Interval approximately 34m. This represents the expected limit of spatial resolution and the CDP bin size should not be larger than 17m.
Source Interval
The limit lateral resolution will be half the dominant wavelength. The dominant frequency can be measured directly from the seismic section, thus simple guideline equation for Source Interval is :
S = Vi / Fdom
Or crossline bin size, ΔY = Vi / (2*Fdom)
Where S = Source interval, Vi is interval velocity and Fdom is frequency dominant. Example : interval velocity 2150 m/s and Fdom 40 Hz, then we have the source interval is 54m. This represents the expected limit of spatial resolution in the crossline direction and the CDP bin size should not be larger than 27m.
Bin size recommendation
The factor which controls bin size in this case is the lateral resolution of the data since structural dips are relatively benign.
Analysis of the geophysical data available indicates that the maximum achievable lateral resolution will be in the order of 17mx27m The CDP bin size should therefore not be larger than this.
Offset distributions and fold requirements
Fold considerations
If the legacy 2D data is 60 fold and is of variable quality. Generally it ranges from fair to good but it is adequate for structural interpretation of major faults and structures. Data of similar quality would be inadequate for detailed structural and stratigraphic interpretation. Therefore higher fold is required to stand a fair chance of gaining adequate signal to noise ratios for detailed reservoir mapping and modelling. The design should therefore allow fold to be as high at the top of the target as the legacy 2D data and to continue to build up through to the base of the target zone.
Common practices calculation the required 3D fold is show below :
3D Fold = 50% - 65% of 2D Fold.
Offset
Minimum Offset
The minimum offset requirement is often calculated from the shallowest objective. The specified shallowest objective,. The most efficient survey design should be with source and receiver lines that capture the shallowest objective or less. This implies would provide high density shots and receivers. Since it will cost so much, we have to make sure the shallowest offset include in the design with sufficient fold number.
Very wide spaced receiver and source lines would also increase the footprint in the data. Therefore the shallowest objective does not form a vital parameter in this case as the candidate designs comfortably satisfy this requirement.
Maximum Offset
The possibility that AVO effects may be present in the deeper target implies that data must be acquired at offsets long enough to give near critical angle reflections as allowed by CDP and stretch muting at the deepest objective. There must also be regularly spaced near offset data so that AVO effects can be estimated robustly if they are present.
Therefore, fold acquired by the top of target must be sufficient to be able to image the stratigraphy and structures and fold must continue to build up until the base of the target zone so that AVO effects at the base of target can be investigated.
The far offset required for AVO analysis can be calculated as :
Far offset = 2 * Z * tan(i)
Where, Z is depth of deepest target and i is the incident angle close to critical angle for the deepest target.
Beside AVO consideration, the largest maximum offset is controlled by NMO Stretch. The maximum offset demonstrated by Andreas Stark, 2008, as a simple derivation as shown below :
ΔT NMO = Tx – To = [(X2/V2) + To2 ]1/2 – To
A stretch of more than Stretching factor, S, would distort the signal after stacking and need to be mute out. This computation allow us to set the maximum spread length. If we only allow 50% stretch at the far offset, we can compute the far offset as follows :
ΔT / To = S
S is stretching factor (%), then :
S. To = ΔT
S. To = [(X2/V2) + To2 ]1/2 - To
(S + 1). To = [(X2/V2) + To2 ] 1/2
(S + 1)2. To2 = (X2/V2) + To2
[(S + 1)2 ) – 1 ]. To2 = (X2/V2)
X2 = To2 V2 . [(S + 1)2 ) – 1 ]
X = To.V [(S + 1)2 ) – 1 ] 1/2
X = To.V [(S2 + 2S)] 1/2
X = Z. [(S2 + 2S)] 1/2
Example, the deep target depth is 2,200 m we can find mute offset of 50% is 2,460m. It means, all traces with far offset more than 2,460m will be cut out under stretch mute 50%.
Azimuth Distribution
A Wide azimuth surveys are desirable for future anisotropy and azimuth dependent processing. However, wide azimuth surveys also tend to have a bias towards longer offset data and generally have more footprint problems than narrow azimuth surveys as they are typically recorded with larger receiver and source line intervals. The footprint is derived from differences in data offset and azimuth properties of the traces from CDP bin to CDP bin and is more acute in low fold, wide azimuth land surveys. CDP stretch and variations in fold at time slice and horizon times, as well as offset properties of the CDP contribute to this effect.
Additionally, surveys which are rich in both azimuths and offsets are costly. Some compromise between the desire to record near offset data and to record all azimuths cheaply needs to be reached in order for the survey acquisition costs to be controlled. The design proposed in this report attempt to combine optimum fold and offset distributions.
Migration Aperture
Migration process is to place dipping horizons and faults into their proper perspective dipping horizons and also the distance required to capture diffraction energy up to take off particular angle from the edge.
Lateral Migration
The distance that a dipping reflector moves during the migration process is give by the equation
d = Z tan(θ),
where d is the distance moved or migration aperture, Z is the depth of the reflector and θ is the angle of dip.
The steepest dipping event expected in the survey area is approximately 30 degrees; however, the migration aperture must be large enough to migrate diffractions, which will arise at discontinuities, bed truncations and faults
The area covered by survey must be extended to account for the aperture and can add significant cost to a survey. This can be more efficiently handled with the use of tail spreads since it is the deeper data that has the larger aperture. Tail spreads provide a migration friendly taper at the edge of the survey and helps to reduce the shot hole drilling effort.
Resolution
Resolution in seismic data is the ability to distinguish between objects, that is, to see a second object in the presence of another.
Vertical Resolution
Vertical resolution relates to how far apart two interfaces must be to distinguish separate reflections from them or how thick a bed must be to allow distinguishable reflections from the bed's top and bottom. The length (in time) of seismic wavelets produces confusion because successive reflections overlap, so it is desirable that the source wavelet be short. The wavelet should have a distinctive, sharp peak to be timed. Side lobes are undesirable because they can be mistaken for true reflections. They add to confusion because of interference. Constructive interference produces an amplitude buildup known as the tuning effect. The tuning thickness is usually taken as the limit of resolution or limit of separability. The vertical resolution equal with one-fourth wavelength :
Limit of Vertical Resolution = Wavelength/4 or,
Limit of Vertical Resolution = V-average/(4*Fdom)
Example, using the equation above we can calculate the vertical resolution for both shallow and deep target as follows :
Shallow target
Shallow target
V-average = 2150 m/s
F dom = 40 Hz
Resolution = 13 m
Deep Target
V-average = 2900 m/s
F dom = 20 Hz
Resolution = 36 m
The calculation above recommend that the limit of layer thickness which can be observed for shallow target is minimum of 13m and for deep target is 36m.
Lateral Resolution
The waves giving rise to a reflection event are reflected from a fairly large, roughly circular area of the reflecting interface known as the first Fresnel zone. Reflections from this zone arrive at a geophone so as to constructively interfere. The radius of this zone is often taken as the horizontal resolution for unmigrated seismic data and to be formulated as follows :
RF = ( V-avg/2) * (T/F)1/2 or
DF = V-avg * (T/F)1/2
Where RF is radius of Fresnel Zone and DF is diameter of Fresnel Zone.
Migration effectively collapses the in-line aspect of the Fresnel zone, so this measure of resolution is not appropriate with the migrated seismic data that are usually used for interpretation. In principle, after migration, lateral resolution is reduced to trace spacing.
Example, using the equation above, then we can calculate the Diameter of Fresnel Zone (DF) before migration on every target horizon as :
Shallow target
V-average = 2150 m/s
F dom = 40 Hz
T = 650 ms
DF = 274 m
Deep Target
V-average = 2900 m/s
F dom = 20 Hz
T = 2100 ms
DF = 940 m
The calculation above recommend that the limit of horizontal resolution referred to the shallow target is 274m.
In order to collapse the diffraction hyperbolic up to 70% of migrated energy (or 15o point of diffraction curve), the edge of the survey boundary must be extent with half of deep Fresnel zone diameter, then, 940 : 2 = 470m for unmigrated data, or equal with deep radius of Fresnel Zone.
Fig-4. Anatomy of diffraction curve
Edge Management
Edge management refers to the aspect of 3-D design that specifies the width of the migration apron and the fold taper. The fold taper is the area at the edge the survey where full-fold (or nearly full-fold) at depth has not been reached before migration. Often a small relaxation in the definition of sufficient fold near the edges can significantly reduce the total size of the survey.
The fold taper can easily add 30% to the total 3-D area, even on large surveys. On small surveys, this percentage increases disproportionately and makes small 3-D surveys, which might be intended to cover as little as 2.5 km2, extremely expensive on a cost-per-area basis. The design of the patch (and there-fore fold taper) is often different in the receiver line direction than in the source line direction because dips may change depending on the azimuth, and then the migration apron changes with dip azimuth.
Generally, fold builds up faster in the cross-line direction than in the in-line direction. This is especially true for narrow azimuth designs because the fold taper in the cross-line direction is directly proportional to the cross-line fold. Careful distribution of receivers can build up fold faster for certain geometries. The orientation of the patch with respect to the survey outline can affect the cost of a survey significantly.
Any 3-D survey should be considered as consisting of three zones. The first (innermost) zone is the domain of the interpreter. All traces lying in this zone should be considered full-fold and fully mi-grated. This is the image area the interpreter should limit his examinations to and use as the basis for geological interpretation. The second (middle) zone is a corridor around the innermost (image) zone.
Fig- 5. Three zone acquisition model (theoretical)
Theoretically, the width of this corridor is equal to the migration apron. In this corridor, the seismic processor assembles full-fold stacked traces. Migration moves most of the energy of these traces into the edge of the innermost (image) zone. The third (outermost) zone is a corridor around the middle zone. The width of this zone is the fold taper. In this corridor, the acquisition planner places sources and receivers to ensure full-fold at the start of the middle (full-fold) zone. The term edge management implies the proper design of these three zones. Compromises can and almost always will be made.
Fig-6. Practical edge management
It is not necessary that traces in the middle zone have near offsets. Farther traces contain deep data be-cause of NMO muting, but deep data migrate farther laterally. If the bins near the outside of the second zone contain only far traces, and bins closest to the innermost zone contain more near traces (hence shallow data), a good migrated result on the edge of the innermost (image) zone should result at all depths.
It may also be possible to relax the 30° requirement for very deep data. This concession reduces the size of the migration apron. Traces that are not quite full-fold may be acceptable for bins near the out-side edge of the middle zone
Rule of Thumb: Migration apron is normally chosen as the larger of:
a) The lateral migration movement of each dip in the expected geology, or
b) The distance required to capture diffraction energy coming upwards at a
scattering angle of 30°, or
c) The radius of the first Fresnel zone.
Calculation of Templates
Below is the example of final template 3-D design for orthogonal and brick patterns. The very detail recommendation including the cost estimation are shown below.
Fig-7. Table shows the final recommendation of 3D parameters
FINAL DELIVERY
At the end of the 3-D design, we will provide our client with a complete report and CD, this final delivery will include :
1. Final Report
This document reports the recommendation of some acquisition parameters with a flexibility for client to choose some options of template. Some consideration and data analysis are also included in this report.
2. SPS Files
Beside the final report, we also provide our client a CD containing : RPS (Receiver), SPS (Shot Point) and XPS (Recording geometry) such that it will make easier for client to "replay" in Mesa software or directly uploaded to surveying software and recording instrument. If the RPS and SPS are included with real co-ordinate, we can create 3-D seismic lines overlay to topographic map.
We are very grateful to assist you acquiring the best 3-D seismic data acquisition with high precision, high resolution in acceptable cost.
The Author : Tri Satyo - Sr. Geophysicist